
SAM started its life in the analysis of school mathematics textbooks and continues to be used in this way (for example: Brantlinger, 2011; Dowling, 2013; Dowling & Burke, 2012). It has, though been deployed in a range of other contexts, for example: Bravenboer (2009, policy in HE); Chung (2011, a textual ethnography of literary discourse); Dowling & Brown (in Dowling, 2009, pedagogic strategies and community structure); and Cunningham (2004, school effectiveness) and Whiteman (2007, online fan communities) deployed SAM as one of their strategies in their respective studies. My current doctoral students are deploying and developing SAM in a diverse array of contexts, only one of them in mathematics. Essentially, SAM does not specify the empirical field or the method of data collection or the type of data that it deals with; rather, its specificity does lie is in its approach to analysis.
Mathematics, though, is a good place to start, because mathematical discourse deploys a highly specialized language in terms of both content—specifically mathematical objects and processes and relations—and expression—mathematical symbols. Essentially, mathematics more so than any other discourse, constitutes a formal language, which is very easy to identify (if sometimes somewhat less easy to read). Making the familiar analytic distinction between expression and content in a textual fragment or utterance and scaling these in terms of high or low level of institutionalization (the level of regularity imposed on their use) led to the scheme in the Figure below.
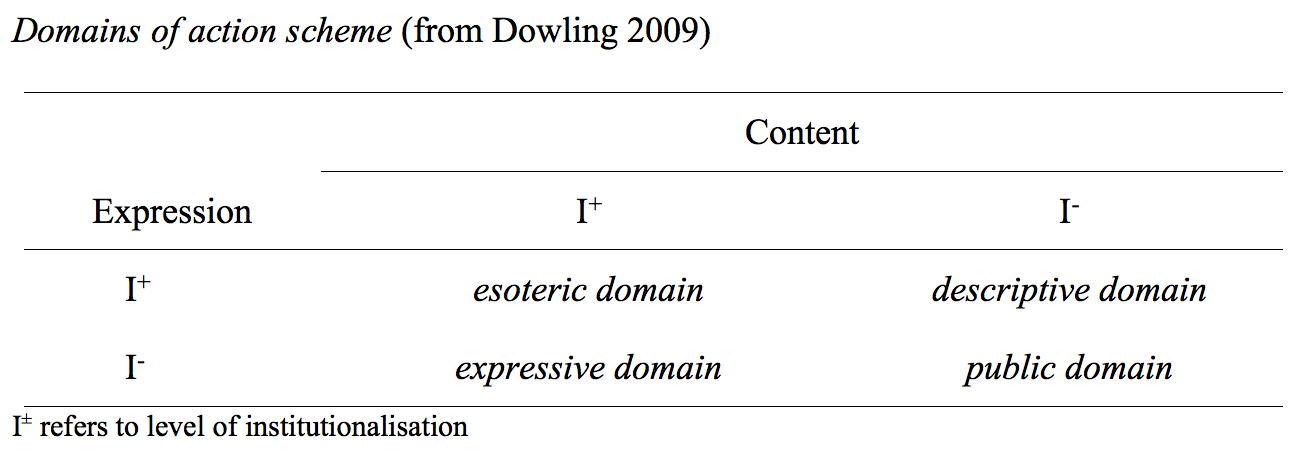
Thinking about mathematics, the esoteric domain is the domain of specialized mathematical content, expressed in mathematical notation. It is only in this domain that mathematics per se can be fully realized. The public domain is the domain of recontextualised, non-mathematical content expressed in non-mathematical notation, generally, everyday language (but sometimes the specialized language of another discourse). The descriptive domain is the domain of mathematical modeling in which non-mathematical objects, processes and relations are
expressed in mathematical terms. The expressive domain is the domain of pedagogic metaphors, such as: a fraction is a piece of cake, an equation is a balance.
The scheme distinguishes between the non-arbitrary and the arbitrary in, in this case, mathematical discourse and enables the analysis of the distribution of data in terms of these domains so that we are able to conclude, for example, that school mathematics texts directed at high and low ‘ability’ school students move between the four domains or are largely restricted to the public domain, respectively. In Dowling (1998) it was also interesting to note that texts dealing with probability, even for high ‘ability’ students also tended to be confined to the public domain (did you ever wonder why you found probability so tricky?). In Dowling (1998) I also revealed the way in which texts directed at high and low ‘ability’ students constructed principles of recognition of their ideal readers in terms of socioeconomic class, so that school mathematics seemed to function as a device for the translation of class into ‘ability’.
The category, recontextualisation, is a central feature of SAM and relates to the way in which one activity regards another, the regarding activity re-organising the latter to bring it into line with its own organizing principles. School mathematics public domain text often concerns domestic activity such as shopping; but its not shopping that we would recognize in Tesco, its shopping that serves the purpose of the mathematics curriculum.
Another key category of SAM is ‘discursive saturation’ (DS±), the extent to which a textual fragment or utterance tends to realize the principles of the active activity—say mathematics—in language. In the analysis of school mathematics texts, it becomes clear that DS+ text is available only to high ‘ability’/social class students.
As I’ve indicated, SAM is not just about mathematics, that’s just where it started/ Chung’s analysis of the ‘crisis discourse’ in literary studies establishes what she refers to as a ‘prosthetic proprioception’ for the activity, recruiting and extending the scheme in the figure above. The proprioception—a term from the expressive domain of her own language—stands as the apparatus that enables literary studies to ‘know’ what it is about and what it is about, it seems, is perpetual and inevitable crisis.
The theoretical background to SAM is rather complex, with key antecedents in the work of Bernstein (although please note, I am NOT a Bernsteinian, despite the fact that he was my own doctoral supervisor—see Dowling, 2009, cc. 4 & 8), Bourdieu, Foucault, Laclau, Lévi-Strauss, Marx, Piaget, Saussure, Wittgenstein, Vygotsky, and many other, inspirational authors. It is, fundamentally, a sociological method and in this respect resonates perhaps more with GT and ethnography than with phenomenology (in any of its realisations). SAM’s concern is with social relations and their production and reproduction in cultural regularities and in action, and this is crystalised in its theoretical primitive:
[T]he sociocultural constitutes and is constituted by strategic, autopoietic action directed at the formation, maintenance and destabilising of alliances and oppositions, the visibility of which, in terms of regularity of practice, is emergent upon the totality of such action and is thereby available for recruitment into subsequent action.
(Dowling, 2013; p. 322)
The ‘domains of action’ scheme, in the figure above, provides one of the ways in which SAM describes regularity of practice in any given activity. The transaction of this primitive with empirical settings generates the kind of scheme presented above: there are currently several dozen such schemes and a list of over 200 specialist terms in the glossary of Dowling (2009). The prevalent form of these schemes is that which is constituted by the Cartesian product of two binary variables. It is frequently suggested that the binary variable is unduly reductionist and that a continuum would be more appropriate. A continuum, however, can only come into being where there is a metric. This is essentially qualitative analysis, so there is no metric and therefore a continuous variable is a mythical desire. I am also accused of putting things into boxes, such as the four domains of the domains of action scheme. This is another misunderstanding. The categories in the boxes are strategies that may be deployed within a given activity. In general, texts that are generated by a particular activity are likely to deploy more than one and often all of the strategies in a given scheme. In analysis, it is necessary to descend to a unit of analysis that exhibits a single strategy. The text as a whole, then, is a composite and its analysis can reveal trajectories and dominant strategies as is illustrated in my observations on mathematics textbooks above. Furthermore, any one scheme produces a commentary from its particular perspective. The development of SAM proceeds via the generation of new schemes as well as the deployment of extant schemes in new contexts. The relationships between the schemes are loose and fluid: the formation of a fully deductive system would render the whole sclerotic, so that, in action, it would be capable of seeing only itself.
DOWLING, P.C. (2013). Social Activity Method: A fractal language for mathematics. Mathematics Education Research Journal. 25(3). pp. 317-340. (Open access, see my website).
BRANTLINGER, A. (2011). ‘Rethinking critical mathematics: a comparative analysis of critical, reform, and traditional geometry instructional texts.’ Educational Studies in Mathematics. 78(3). 395-411.
BRAVENBOER, D. (2009). Commodification and the official discourse of Higher Education. PhD thesis. Intitute of Education, University of London.
CHUNG, S-y. (2011). ‘The modality of the textual institutionalisation of literary studies: towards a sociology.’ Sociological Research Online. 16/3/3.
CUNNINGHAM, R. (2004). An exploration of the potential of complexity theory for addressing the limitations of current models of change and innovation in educational practice. EdD thesis. Intitute of Education, University of London.
DOWLING, P.C. (1998). The Sociology of Mathematics Education: mathematical myths/pedagogic texts. London. Falmer Press.
DOWLING, P.C. (2009a). Sociology as Method: departures from the forensics of culture, text and knowledge. Rotterdam. Sense.
DOWLING, P.C. (2009b).哲学の道. In B. Sriraman & S. Goodchild (Eds). Relatively and Philosophically Earnest. IAP. Pre-publication version available at http://www.pauldowling.me/publications/philosopherswalk.pdf [The published version will have different page numbers etc.].
DOWLING, P.C. (in press). 祇園祭 (Thoughts on purification, liminality, art, fermented shark, mathematics, and education for creativity). In C. Bergsten & B. Sriraman (Eds). Refractions of mathematics education. Festschrift for Eva Jalonka. Charlotte, NC: Information Age Publishing.
DOWLING, P.C. & BURKE, J. (2012). ‘Shall We Do Politics or Learn Some Maths Today? Representing and interrogating social inequality.’ In H. Forgasz & F. Rivera (Eds). Towards Equity in Mathematics Education: gender, culture, and diversity. Heidelberg. Springer. 87-104. Available at www.pauldowling.me/publications/Dowling&Burke.pdf [The published version will have different page numbers etc.].
WHITEMAN, N. (2007). The Establishment, Maintenance and Destabilisation of Fandom: A study of two online communities and an exploration of issues pertaining to internet research. PhD thesis. Intitute of Education, University of London.